Solution to Abstract Algebra by Dummit & Foote 3rd edition Chapter 2.3 Exercise 2.3.6
In $\mathbb{Z}/(48)$, write out all elements of $\langle \overline{a} \rangle$ for every $\overline{a}$. Find all inclusions among subgroups of $\mathbb{Z}/(48)$.
Solution: By Theorem 7 in the text, we know that for every integer $m$, $\langle m \rangle = \langle \mathsf{gcd}(m,48) \rangle$. $$\langle 0 \rangle = \{ \overline{0} \}$$ $$\langle \overline{24} \rangle = \{ \overline{0}, \overline{24} \}$$ $$\langle \overline{16} \rangle, \langle \overline{32} \rangle = \{ \overline{0}, \overline{16}, \overline{32} \}$$ $$\langle \overline{12} \rangle, \langle \overline{36} \rangle = \{ \overline{0}, \overline{12}, \overline{24}, \overline{36} \}$$ $$\langle \overline{8} \rangle, \langle \overline{40} \rangle = \{ \overline{0}, \overline{8}, \overline{16}, \overline{24}, \overline{32}, \overline{40} \}$$ $$\langle \overline{6} \rangle, \langle \overline{18} \rangle, \langle \overline{30} \rangle, \langle \overline{42} \rangle = \{ \overline{0}, \overline{6}, \overline{12}, \overline{18}, \overline{24}, \overline{30}, \overline{36}, \overline{42} \}$$ $$\langle \overline{4} \rangle, \langle \overline{20} \rangle, \langle \overline{28} \rangle, \langle \overline{44} \rangle = \{ \overline{0}, \overline{4}, \overline{8}, \overline{12}, \overline{16}, \overline{20}, \overline{24}, \overline{28}, \overline{32}, \overline{36}, \overline{40}, \overline{44} \}$$ \begin{align*}&\ \langle \overline{3} \rangle, \langle \overline{9} \rangle, \langle \overline{15} \rangle, \langle \overline{21} \rangle, \langle \overline{27} \rangle, \langle \overline{33} \rangle, \langle \overline{39} \rangle, \langle 45 \rangle \\=&\ \{ \overline{0}, \overline{3}, \overline{6}, \overline{9}, \overline{12}, \overline{15}, \overline{18}, \overline{21}, \overline{24}, \overline{27}, \overline{30}, \overline{33}, \overline{36}, \overline{39}, \overline{42}, \overline{45} \}\end{align*} \begin{align*}&\ \langle \overline{2} \rangle, \langle \overline{10} \rangle, \langle \overline{14} \rangle, \langle \overline{22} \rangle, \langle \overline{26} \rangle, \langle \overline{34} \rangle, \langle \overline{38} \rangle, \langle \overline{46} \rangle \\=&\ \{ \overline{0}, \overline{2}, \overline{4}, \overline{6}, \overline{8}, \overline{10}, \overline{12}, \overline{14}, \overline{16}, \overline{18}, \overline{20}, \overline{22}, \overline{24}, \overline{26}, \overline{28}, \overline{30}, \overline{32}, \overline{34}, \overline{36}, \overline{38}, \overline{40}, \overline{42}, \overline{44}, \overline{46} \}\end{align*} \begin{align*}&\ \langle 1 \rangle, \langle 5 \rangle, \langle 7 \rangle, \langle 11 \rangle, \langle 13 \rangle, \langle 17 \rangle, \langle 19 \rangle, \langle 23 \rangle, \langle 25 \rangle, \\ &\ \langle 29 \rangle, \langle 31 \rangle, \langle 35 \rangle, \langle 37 \rangle, \langle 41 \rangle, \langle 43 \rangle, \langle 47 \rangle = \mathbb{Z}/(48)\end{align*}
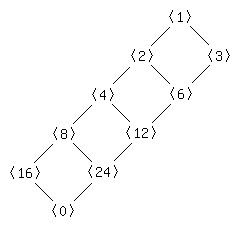
We visualize the containments among these subgroups as in the following diagram. Where two subgroups are connected by a line, the lower is contained directly in the higher; intermediate containments are not shown.